Compound Interest refers to the accumulated growth of an investment, with interest calculated on the principal amount plus interest on any growth that occurred since the investment began.
In the world of investments and loans, the principal amount is the original amount. If you originally invested $100, and now it is worth $160, the principal amount is $100 (the amount you invested when you started).
Compound interest plays a crucial role in the growth of investment, savings, and loans over time.
The University of Cincinnati has the following definition of ‘compound interest’:
“The amount of interest earned on a loan or deposit based on the initial amount plus the interest accumulated over time.”
Compound interest vs. simple interest
Unlike simple interest, which is calculated only on the principal amount, compound interest calculates interest on both the initial principal and also the accumulated interest from previous periods.
Compound interest is “interest on interest.”
With each period growing at a compounding rate, your investment grows at an accelerating rate.
Significant growth over time
Compound interest can lead to a significant interest in the total amount over time, especially if you invest over a long period.
The rate at which your investment grows depends on:
- The principal amount.
- The interest rate.
- The frequency of compounding, that is, how often interest is added to the principal.
Compound Interest Calculator
[compound_interest_calculator]
An example of compound interest
Let’s say you invested $1,000 and you earned an annual interest rate of 5%, which is compounded annually.
After one year you would earn $50 in interest, so your total would increase to $1,050.
In the second year, you would earn interest on the whole amount you have so far, not just the principal. So, you’d have to calculate 5% of $1,050, which is $52.5, and add that to $1,050, which would give you $1102.5.
This process of adding interest to the principal and the increased amounts each year can increase the size of your investment over time.
After 10 years, your $1,000 would be worth $1,628.89. You would have earned $628.89 in interest alone.
If you had earned 10% each year, your initial $1,000 investment would be worth $2,593.74 after ten years
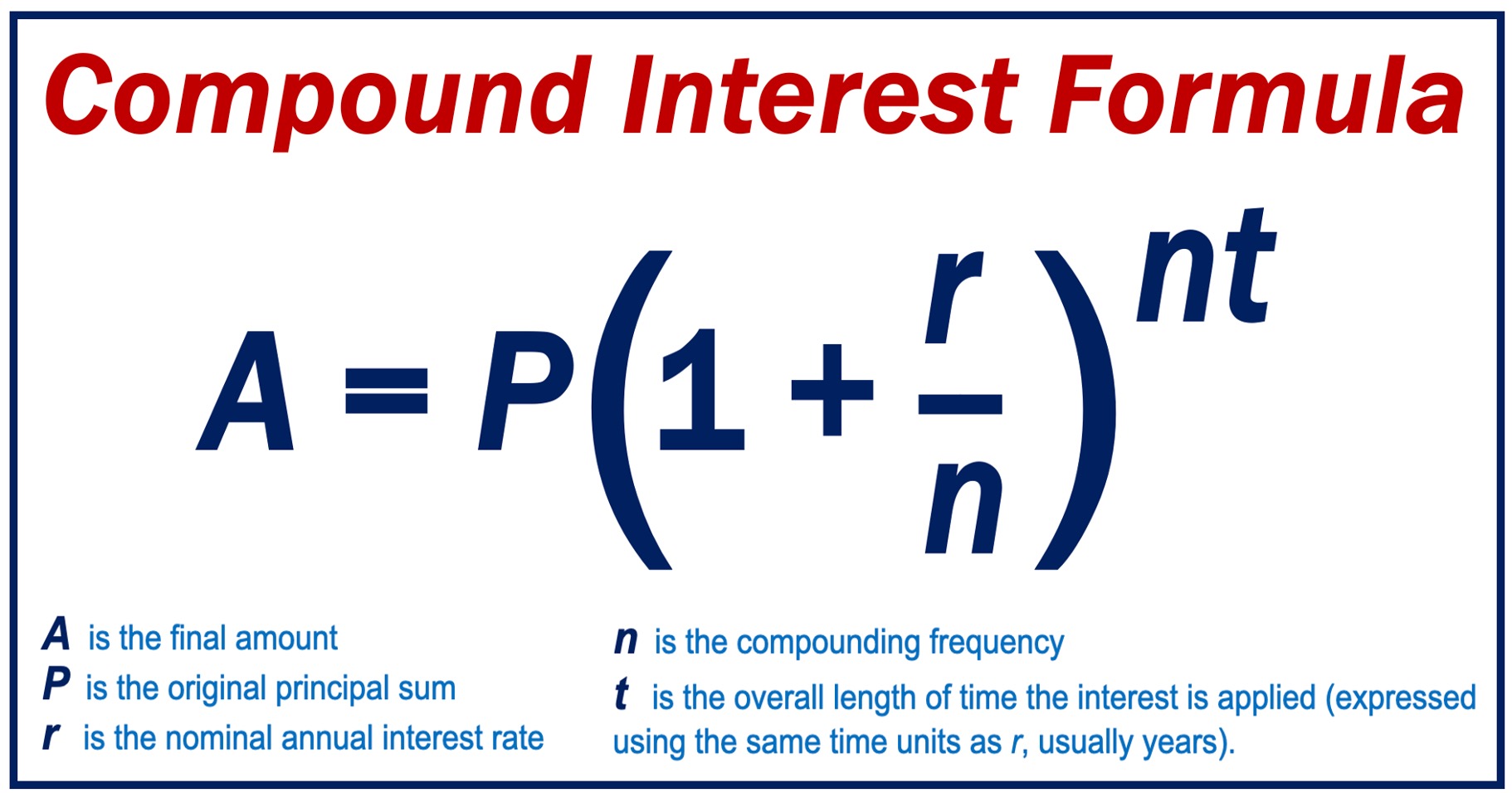
Compound interest formula
The formula below allows us to determine the amount of interest earned on an investment/savings over time, taking into account the compounding effect:
Start saving early
The effect of compound interest on investment growth becomes more apparent over long periods. That is why we should start saving and investing early in life.
If you start saving in your early 20s, your investment will be worth a lot of money by the time you retire.
The frequency of compounding varies. Interest on some investments occurs annually, while in others it may be daily, monthly, or quarterly.
