A team of Polish researchers warns that a giant financial meltdown will probably occur in about a decade. Their multifractal analyses of the main stock market index warn that it is time for a hyper financial meltdown. Put simply; they predict that the outlook for the global economy from the mid-2020s onward looks extremely bleak.
Scientists from the Institute of Nuclear Physics of the Polish Academy of Sciences in Cracow wrote about their study and findings in the journal Complexity (citation below). The authors were Stanisław Drożdż, Rafał Kowalski, Paweł Oświȩcimka, Rafał Rak, and Robert Gȩbarowski.
Their pessimistic forecast is the result of an advanced statistical analysis of the S&P 500 stock market index.
The S&P 500 or Standard and Poor’s 500 is a stock market index that includes the 500 biggest companies listed in NASDAQ and the NYSE. NYSE stands for New York Stock Exchange. S&P measures size according to companies’ total market capitalization.
In about a decade, there will be a giant financial meltdown such as never before, say the researchers. They also say that we still have a chance to save the world.
Financial meltdown will make others look small
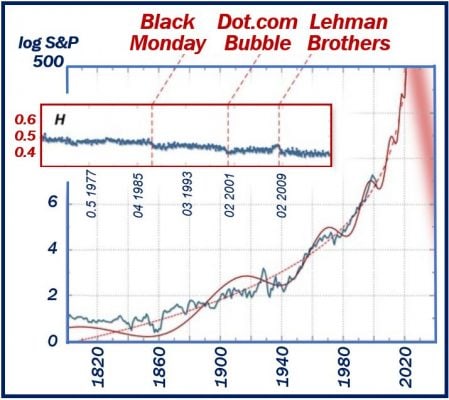
The bankruptcy of Lehman Brothers, the dot.com bubble, and Black Monday were events that shook the world economy.
However, soon we may have to deal with such a colossal collapse of financial markets that previous crashes will seem like minor stumbling blocks in comparison.
Prof. Stanisław Drozdz (IFJ PAN, Cracow University of Technology) said:
“The data is, unfortunately, quite unambiguous. It seems that since the mid-2020s, a global financial crash of a previously unprecedented scale is highly probable. This time the change will be qualitative. Indeed radical!”
Scientists from the Henryk Niewodniczanski Institute of Nuclear Physics (IFJ PAN) gathered and analyzed various economic data. The data included the daily listing of the S&P 500 index spanning from January 1950 to December 2016.
Aim was not to predict a financial meltdown
According to an IFJ PAN press release:
“The main goal of the Cracow researchers’ article was not to make catastrophic forecasts, but to credibly present issues related to the occurrence of multifractal effects (i.e. those in which in order to see self-similarity, different fragments of the structure under investigation have to be increased at different rates) in the financial time series (i.e. prices or stock market indices).”
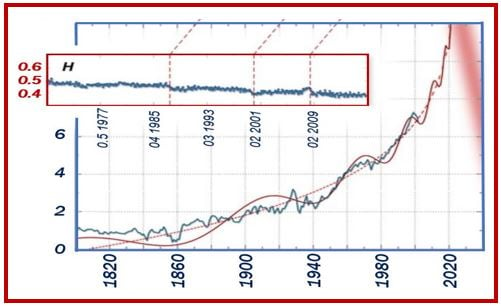
A graph grabbed the researchers’ attention. It showed changes in the Hurst exponent, calculated for S&P 500 based on multifractal spectra obtained during analysis.
A fractal is a never-ending pattern. Fractals are patterns that are infinitely complex – they are self-similar across different scales. Fractals are created by repeating a simple process again and again in a never-ending feedback loop. Multifractal means relating to or consisting of many fractals.
The Hurst exponent
The Hurst exponent may assume values from zero to one. It reflects how susceptible a system is to a change in trend.
When value = 0.5
A value of, for example, 0.5 means the market could just as easily go up as down.
When value = <0.5
A value below 0.5 indicates a probability of a decline or vice-versa. In a context of financial markets, we can interpret it as a symptom of nervousness.
When value = >0.5
Any value above 0.5 indicates the persistent nature of changes, and also the tendency of the system to be building a trend. In other words, what just happened will probably happen next time. After an increase, there is subsequently a greater probability of another rise, and after a fall, there is a greater probability of another decrease.
Stable mature markets
The Hurst exponent of stable, mature markets is equal to 0.5. They may deviate from 0.5, but never by much.
On October 19th, 1987, the Hurst graph for the S&P 500 started at 0.5. However, the famous Black Monday – a crash – occurred.
After Black Monday, the exponent decreased slightly . However, for the next decade or so, it remained relatively stable.
At the turn of this century, there was a clear decline. By March 2000, the dot.com bubble had popped. As it did before, the Hurst exponent subsequently stabilized again. However, this time for a shorter period.
During the second half of this century’s first decade, it suddenly started growing rapidly, only to fall in September 2008 when Lehman Brothers went bankrupt.
Since Lehman Brothers’ collapse, the Hurst exponent has not only failed to return to 0.5 but during the last decade, it has quite clearly and systematically remained below 0.4. The researchers describe this value as ‘particularly worrying.’
Growing nervousness in the global market
Prof. Drozdz noted:
“What is also striking in the changes in the Hurst exponent for the S&P 500 is the shortening time intervals between consecutive crashes and the fact that after each collapse the indicator never returns to its original level.”
“We have a clear signal here that the nervousness of the world market is growing all the time, for decades, regardless of changing people, business entities or technology.”
Prof. Drozdz added:
“The thing is that analogous self-similar dependence can also work on larger time scales. In which case, all previous crashes would only be precursors of a much larger and more dangerous event. When we come across a process with similar dynamics in physics, we talk about phase transition of the second type, such as the appearance or disappearance of magnetic properties in magnetic material around the Curie temperature.”
The credibility of such a bleak forecast remains an open question. If in the coming years, financial markets do not change qualitatively, there is a chance that the worst-case scenario of the development of events really happens.
The worlds of financial markets and physics
The researchers add that we must bear in mind that there is a significant difference between the worlds of financial markets and physics/mathematics.
Mathematical models and laws constructed within physics are relatively complicated and effective. Financial markets, on the other hand, are significantly more complex. Financial market participants remember, learn, and can react both emotionally and logically, i.e., they are changeable.
In a press release, IFJ PAN wrote:
“There is no shortage of examples proving that when knowledge about a law with the power to forecast is disseminated among a significant number of market participants, the market changes rapidly and the detected regularity disappears. Will the same happen in the case of the impending hyper-crash?”
What could prevent a financial meltdown? Nobody is sure
The problem is that nobody knows what or how something would have to affect the global market to stop an impending collapse from happening. The emerging markets of cryptocurrencies, for example, might be one remedy. However, nobody knows whether they will be.
Who knows whether it would be possible to introduce the necessary changes in just a few years? Unfortunately, the researchers add, we do not have a longer period at our disposal.
From the mid-2020s onward, the future of the world economy looks very bleak. The threat of a financial meltdown will get bigger and bigger.
Prof. Drozdz said:
“Probably, we are the only ones who can’t lose on this forecast. If the hyper-crash does occur, we will have shown the power of our multifractal statistical tools in a spectacular way.”
“Personally, however, I would prefer for this not to happen. If this is the case and the hyper-crash does not occur, we will still have the quite acceptable interpretation that our forecast was… correct, but today’s press release will have influenced the behavior of market participants and, well, we have just saved the world!”
Citation
“Dynamical Variety of Shapes in Financial Multifractality,” Stanisław Drożdż, Rafał Kowalski, Paweł Oświȩcimka, Rafał Rak, and Robert Gȩbarowski. Complexity Volume 2018, Article ID 7015721, 13 pages. DOI: https://doi.org/10.1155/2018/7015721.